Order of Operations Printable Worksheet: Master the PEMDAS Hierarchy
Mathematics, the language of the universe, demands precision and adherence to rules. One such crucial rule is the Order of Operations, a guiding principle that dictates the sequence in which mathematical expressions are evaluated. Understanding this hierarchy is paramount for solving complex equations accurately.
This Order of Operations Printable Worksheet is designed to provide a comprehensive and engaging learning experience, empowering you to conquer mathematical challenges with confidence. Dive into the world of PEMDAS (Parentheses, Exponents, Multiplication, Division, Addition, Subtraction) and witness the transformative power of organized problem-solving.
Order of Operations Overview
Blud, listen up. When you’re sorting out maths problems, there’s a certain way you gotta do it, innit? It’s called the order of operations, or PEMDAS for short.
PEMDAS stands for Parentheses, Exponents, Multiplication and Division, Addition and Subtraction. That means you start with anything inside brackets, then do any powers or roots, then multiply and divide (from left to right), and finally add and subtract (again, from left to right).
Significance of the Order
Why does it matter? Well, if you don’t follow PEMDAS, you’ll get the wrong answer. For example, if you add 3 and 4 before you multiply by 2, you’ll get 14 instead of 14. So, always stick to PEMDAS, yeah?
Printable Worksheet Design
Make your worksheet visually appealing and engaging to grab students’ attention. Use bright colors, fun fonts, and eye-catching graphics. Make sure the layout is clear and easy to follow, with ample space for students to write their answers.
Include clear instructions and examples for each problem. This will help students understand what they need to do and how to approach the problems. You can also provide worked-out examples to show students how to solve similar problems.
Use Engaging Content
Incorporate real-world examples and relatable scenarios to make the worksheet more engaging. This will help students connect the concepts to their own lives and make the learning process more meaningful.
Practice Problems
Yo, blud! Let’s get stuck into some practice probs to smash this order of operations malarky.
Numeric Expressions
These are like, easy peasy lemon squeezy. Just follow the BODMAS rules and you’ll be golden.
- 2 + 3 × 4 = 14
- (5 – 2) × 3 = 9
- 4 + 5 – 2 = 7
Algebraic Expressions
These are a bit more trixy, but no worries. Just remember the rules and you’ll be sorted.
- 3x + 2y – 5 = 3(2) + 2(1) – 5 = 1
- (x + 2)(x – 1) = x(x) + x(-1) + 2(x) + 2(-1) = x^2 + x – 2x – 2 = x^2 – x – 2
Mixed Expressions
These are a mix of numeric and algebraic expressions. Just use the BODMAS rules and you’ll be sweet.
- 2x + 3y – 4 = 2(3) + 3(2) – 4 = 6 + 6 – 4 = 8
- (2 + 3x) × 4 = (2 + 3(2)) × 4 = (2 + 6) × 4 = 8 × 4 = 32
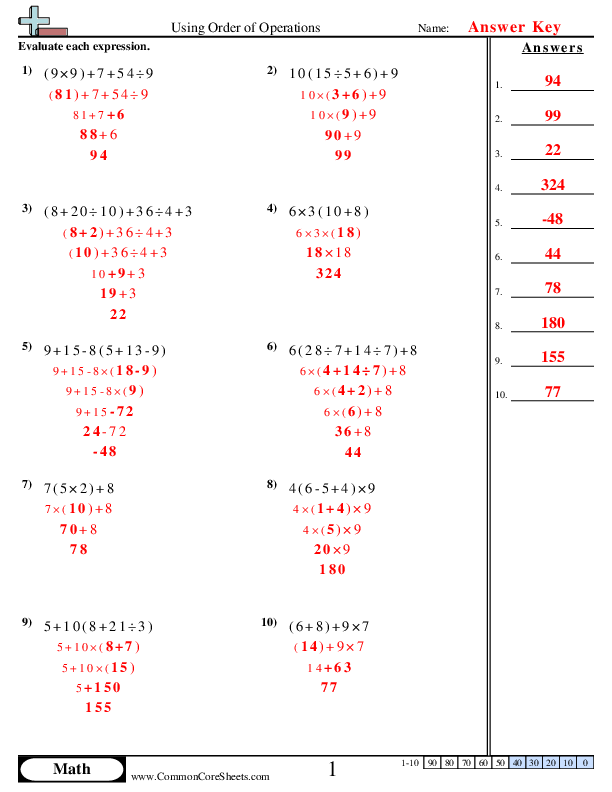
Answer Key
Check your work using the comprehensive answer key provided below. Each step in solving the problems is explained clearly for your understanding.
Step-by-Step Solutions
For each problem, the answer key provides a detailed breakdown of the steps involved in solving it. This includes identifying the order of operations, evaluating parentheses, and performing calculations in the correct order.
Differentiation
To cater to learners of varying abilities, here are some ideas for differentiating the Order of Operations worksheet:
For Struggling Students
- Provide extra scaffolding by breaking down the problems into smaller steps.
- Offer visual aids like number lines or diagrams to support understanding.
- Allow students to use manipulatives or calculators as needed.
For Advanced Learners
- Challenge students with more complex problems involving multiple operations and parentheses.
- Introduce them to algebraic expressions and equations that incorporate the order of operations.
- Encourage them to explore real-world applications of the order of operations, such as in finance or engineering.
Frequently Asked Questions
What is the significance of the Order of Operations?
The Order of Operations ensures consistency in solving mathematical expressions, preventing ambiguity and ensuring accurate results.
How can I differentiate the worksheet for students with varying abilities?
Provide simpler problems for struggling students, while challenging advanced learners with complex expressions or word problems.
What is the purpose of the Answer Key?
The Answer Key allows students to self-check their work, identify errors, and reinforce their understanding of the Order of Operations.