Multiplying Fractions Printable Worksheet: A Comprehensive Guide for Students
Multiplying fractions is a fundamental mathematical operation that requires a thorough understanding of fraction concepts. Printable worksheets serve as valuable tools for students to practice and reinforce their knowledge of this essential skill. This guide will delve into the significance of printable worksheets for multiplying fractions, providing a step-by-step guide to the process, highlighting common mistakes to avoid, and showcasing real-world applications.
In addition, this guide will offer a comprehensive list of frequently asked questions (FAQs) to address common queries related to multiplying fractions. By utilizing printable worksheets and following the strategies Artikeld in this guide, students can enhance their proficiency in fraction multiplication and develop a strong foundation for mathematical problem-solving.
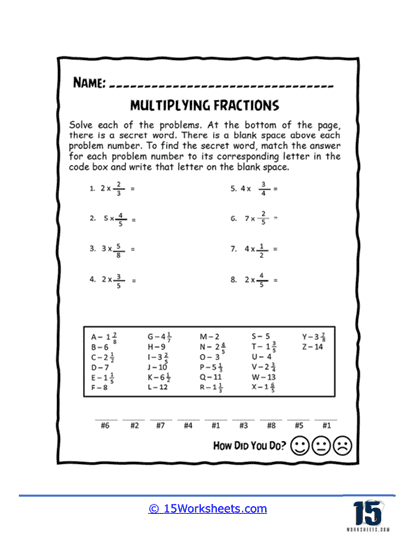
Printable Worksheet on Multiplying Fractions
Multiplying fractions is a fundamental skill in mathematics. A printable worksheet provides students with a structured and convenient way to practice this skill, allowing them to work at their own pace and reinforce their understanding.
Benefits of Using a Printable Worksheet for Multiplying Fractions
* Provides Ample Practice: A worksheet offers numerous problems for students to solve, giving them ample opportunities to practice multiplying fractions and develop proficiency.
* Encourages Independent Learning: Worksheets allow students to work independently, freeing up the teacher for other tasks. They can review the instructions, solve the problems, and check their answers at their own pace.
* Assesses Understanding: Worksheets can be used as formative assessments to gauge students’ comprehension of fraction multiplication. By analyzing their responses, teachers can identify areas where students need additional support.
Tips for Creating an Effective Printable Worksheet on Multiplying Fractions
* Clear Instructions: Provide clear and concise instructions that explain the steps involved in multiplying fractions. Include examples to illustrate the process.
* Variety of Problems: Offer a variety of problems to cater to different levels of understanding. Include problems with whole numbers, mixed numbers, and improper fractions.
* Answer Key: Provide an answer key so that students can check their work and identify any errors. This helps them learn from their mistakes and improve their accuracy.
* Visual Aids: Consider incorporating visual aids such as diagrams or number lines to make the concepts more accessible to students.
Step-by-Step Guide to Multiplying Fractions
Multiplying fractions is a skill that comes in handy in various everyday situations. Follow this simple guide to master the technique:
### Multiplying the Numerators and the Denominators
The first step involves multiplying the numerators of the fractions. For instance, if you want to multiply 1/2 by 2/3, you multiply 1 and 2 to get 2.
Next, multiply the denominators of the fractions. In this case, multiply 2 and 3 to get 6.
### Writing the Product
The product of the fractions is the new fraction obtained by writing the product of the numerators over the product of the denominators. In our example, the product is 2/6, which can be simplified to 1/3.
### Example
Let’s multiply 3/4 by 5/6:
– Multiply the numerators: 3 × 5 = 15
– Multiply the denominators: 4 × 6 = 24
– Write the product: 15/24
– Simplify the fraction: 15/24 = 5/8
So, 3/4 multiplied by 5/6 equals 5/8.
Common Mistakes in Multiplying Fractions
Multiplying fractions can be tricky, and students often make mistakes. Here are some of the most common mistakes and how to avoid them.
One of the most common mistakes is forgetting to multiply the numerators and denominators of the fractions. For example, a student might multiply 1/2 by 3/4 and get 3/8. However, the correct answer is 3/8.
Another common mistake is multiplying the numerators and denominators of the fractions in the wrong order. For example, a student might multiply 1/2 by 3/4 and get 4/6. However, the correct answer is 3/8.
Finally, some students make the mistake of simplifying the fraction before multiplying it. For example, a student might multiply 1/2 by 3/4 and get 3/8. However, the correct answer is 3/8.
Tips for Avoiding Mistakes
- Be careful to multiply the numerators and denominators of the fractions correctly.
- Don’t forget to simplify the fraction after multiplying it.
- Practice multiplying fractions regularly.
Examples of Multiplying Fractions
Multiplying fractions is a fundamental operation in mathematics that involves finding the product of two or more fractions. Understanding how to multiply fractions is crucial for solving various mathematical problems and real-world applications.
To multiply fractions, we follow a simple rule: multiply the numerators (top numbers) of the fractions and multiply the denominators (bottom numbers) of the fractions. The resulting fraction is the product.
Multiplying Proper Fractions
Let’s consider multiplying two proper fractions, where the numerator is less than the denominator:
* Example 1: Multiply 1/2 by 1/3.
“`
1/2 × 1/3 = (1 × 1) / (2 × 3) = 1/6
“`
* Example 2: Multiply 3/4 by 2/5.
“`
3/4 × 2/5 = (3 × 2) / (4 × 5) = 6/20 = 3/10
“`
Multiplying Improper Fractions
An improper fraction has a numerator that is greater than or equal to the denominator. When multiplying improper fractions, we first convert them to mixed numbers (a whole number and a proper fraction) and then multiply.
* Example 1: Multiply 5/3 by 2/3.
Convert 5/3 to a mixed number: 5/3 = 1 2/3
“`
(1 2/3) × 2/3 = (5/3) × (2/3) = 10/9 = 1 1/9
“`
* Example 2: Multiply 7/2 by 3/4.
Convert 7/2 to a mixed number: 7/2 = 3 1/2
“`
(3 1/2) × 3/4 = (7/2) × (3/4) = 21/8 = 2 5/8
“`
Multiplying Mixed Numbers
A mixed number consists of a whole number and a proper fraction. To multiply mixed numbers, we first convert them to improper fractions and then multiply.
* Example 1: Multiply 1 1/2 by 2 1/3.
Convert 1 1/2 to an improper fraction: 1 1/2 = 3/2
Convert 2 1/3 to an improper fraction: 2 1/3 = 7/3
“`
(3/2) × (7/3) = 21/6 = 3 3/6 = 3 1/2
“`
* Example 2: Multiply 3 2/5 by 1 3/4.
Convert 3 2/5 to an improper fraction: 3 2/5 = 17/5
Convert 1 3/4 to an improper fraction: 1 3/4 = 7/4
“`
(17/5) × (7/4) = 119/20 = 5 19/20
“`
Applications of Multiplying Fractions
Multiplying fractions finds numerous applications in real-world scenarios. From cooking to construction to scientific calculations, fractions play a crucial role in solving problems and making accurate measurements.
In Cooking
When baking or cooking, precise measurements are essential for successful results. Multiplying fractions allows you to scale recipes up or down, adjust ingredient quantities, and ensure the correct proportions for a balanced dish.
In Construction
Construction workers often encounter fractions when measuring materials, calculating angles, and determining the dimensions of structures. Multiplying fractions helps them calculate the exact amounts of materials needed, ensuring the stability and safety of buildings.
In Science
In scientific fields like physics and chemistry, fractions are used to represent ratios, concentrations, and probabilities. Multiplying fractions enables scientists to perform calculations involving these quantities, analyze data, and draw accurate conclusions.
Additional Resources for Multiplying Fractions
Websites
- Math is Fun: Provides interactive lessons, practice exercises, and quizzes on multiplying fractions. Website
- Khan Academy: Offers video tutorials, step-by-step instructions, and practice problems on multiplying fractions. Website
- Coolmath4kids: Presents interactive games, puzzles, and activities to help students learn and practice multiplying fractions. Website
Videos
- Multiplying Fractions – Animated Lesson: A clear and engaging animated video that explains the steps for multiplying fractions. Video
- Multiplying Fractions – Step-by-Step Tutorial: A detailed video tutorial that guides students through each step of multiplying fractions. Video
- Multiplying Fractions – Practice Problems: A video that provides practice problems on multiplying fractions with solutions and explanations. Video
Interactive Games
- Fraction Multiplication War: A fun and interactive game where students play cards to practice multiplying fractions. Game
- Fraction Multiplication Match-Up: A game that requires students to match fractions to their products. Game
- Fraction Multiplication Bingo: A bingo game that helps students practice multiplying fractions and identify equivalent fractions. Game
Questions and Answers
Why are printable worksheets beneficial for practicing fraction multiplication?
Printable worksheets provide students with a structured and organized way to practice multiplying fractions. They allow students to work at their own pace, repeat problems for reinforcement, and receive immediate feedback on their answers.
What are some common mistakes students make when multiplying fractions?
Common mistakes include multiplying the numerators and denominators directly, forgetting to simplify the result, and incorrectly converting mixed numbers to improper fractions.
How can I create an effective printable worksheet for multiplying fractions?
When creating a printable worksheet, consider including a variety of problem types, such as multiplying proper fractions, improper fractions, and mixed numbers. Provide clear instructions and examples, and offer answer keys for self-assessment.
What are some real-world applications of multiplying fractions?
Multiplying fractions is used in various fields, including cooking (scaling recipes), construction (calculating materials), and science (converting units of measurement).